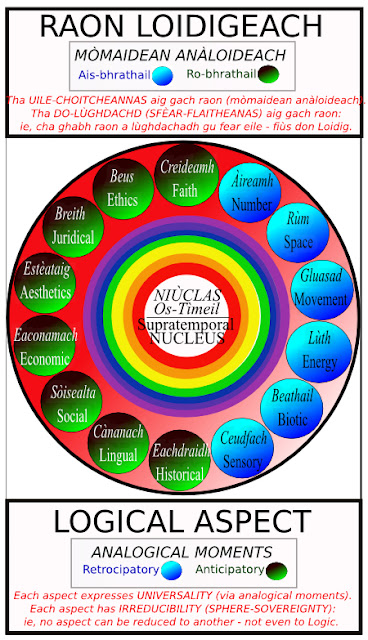
The logicistic concept of 'Dimension überhaupt' (dimension in general), and the modal shift of meaning in this pseudo-concept.
__________________
Het logicistisch begrip der ‘Dimension überhaupt’ en de modale zin-verschuiving in dit pseudo-begrip.
__________________
Coincheap loidigisteach 'Dimension überhaupt' (tomhas san fharsaingeachd) agus carachadh-cèille modalach sa choincheap-brèige seo.
__________________
Click image to enlarge
The logicistic shifts of meaning in NATORP's attempt to derive the so-called imaginary function of number from the fundamental logical relation of isolation and unification may be called palpable. The logicistic principle of the origin does not allow theoretical thought to respect the modal boundaries of meaning between number, space and logical analysis. That is why the logical continuity and direction of the movement of thought, as spatial and kinematic analogies, have to do duty as a basis for the inclusion into the number-concept of the moments of continuity and dimension as original logical moments of meaning, without any reference to the original modal sense of space. The multiplicative relations of the plus- and minus-directions in the modal temporal order of number, which have the meaning of discrete quantity, are interpreted as dimensions. After assuming actual continuity in the basic series NATORP seems really to have derived the concept of dimension from the 'logical' meaning of number itself. But this concept of dimension has become a pseudo-logical general notion without any modal definition of its meaning. This is, moreover, proved by NATORP's thesis that in order to be able to think the dimensions of space, it is necessary first to know how to think the Dimensionen überhaupt' (dimensions in general) (1).
_________________
(1) Die logischen Grundlagen, p. 263.
_________________
The concept: 'Dimension überhaupt' has been obtained in a logicistic way from the logical analogy of dimension [space]. But the fact has been overlooked that this analogy cannot exist without its meaning-substratum in the original modal meaning of space.
It is, however, very instructive for our insight into the complex structure of the spatial anticipations within the original meaning-aspect of number that this logicism does not see its way to include the moment of dimension into the number-concept without first introducing extensive continuity into the series of the real numbers.
Complex systems of number and the theory of groups. The formalistic conception of the symbol i.
In the complex modal functions of number there is no question of a mere anticipation of spatial dimensions. Rather they anticipate, via these dimensions, modal directions of pure movement, and they do so under the guidance of the movement of theoretical thought.
This holds good both for the system of the so-called ordinary complex functions of number (A + Bi etc.), and for the systematic extensions into the systems of the so-called quaternions, biquaternions and triquaternions. The modern inclusion of the whole of the theory of the complex functions of number into the so-called theory of groups (2) which investigates the invariant relations in the transformations within the group, only emphasizes this state of affairs which is revealed to us by the structural analysis of the meaning-aspect of number.
____________________
(2) Cf. Dr J. WOLFF, Complexe Getallenstelsels (1917, Groningen), p. 15 ff.
____________________
Every system of complex numbers is supposed to refer to two interchangeable groups of linear homogeneous transformation, and vice versa. As to this supposition, the intermodal coherence of meaning of the complex function of number with the modal structure of the spatial dimensions and the pure directions of movement cannot he philosophically irrelevant.
In the quaternion-systems consisting of one real and three imaginary units (i, j, k) (3), the absence of the so-called commutative quality of multiplication (entitling us in ordinary algebra to change the product ab into that of ba) cannot be understood unless its connection with the directions of pure movement is taken into account; the quaternion anticipates the latter in the meaning-aspect of number.
__________________
(3) i, j and k are then interpreted as rotations of 90° round three axes, placed vertically on each other, so that their squares are — 1.
__________________
The formalistic trend in mathematics erroneously hold the imaginary unit i to be a self-sufficient abstract construction of thought with an unexplained meaning, while it is assumed to be immaterial that this unit i can be adequately represented in a sensory spatial picture of motion. In our treatment of the modal subject-object relation it will appear that a sensory representation pre-supposes a sensory original, and that a non-sensory original can never be depicted in a sensory way.
The point at issue is much more concerned with an (intrinsically cosmological) intermodal coherence of meaning into which the complex function of number has been fitted according to its modal structure. Even nominalistic formalism has to reckon with this, at least implicitly, in its supposedly arbitrary definitions if it is at all to be able to fix the complex functions of number in the theoretical vision (4).
__________________
(4) From a formalistic standpoint HANKEL remarks (in his Theorie der complexen Zahlensysteme (1867) p. 66) on the symbol i: 'It is merely a sign for an imaginary mental object which is called the imaginary unit, whose nature, however, in pure theory remains entirely undetermined and must remain so, as in this we have only to do with its formal relations and complications' ["es ist weiter nichts als ein Zeichen für ein eingebildetes mentales Objekt, welches man die imaginäre Einheit nennt, dessen eigentliches Wesen aber in der reinen Theorie ganz unbestimmt bleibt und unbestimmt bleiben musz, da wir uns in dieser nur mit seinen formalen Verknüpfungen zu beschäftigen haben."] But the imaginary function has not a hidden 'metaphysical nature' as HANKEL thinks. Its functional side itself is at issue, on which its entire complex of apriori law-conformable relations depend!
__________________
Click image to enlarge
Het logicistisch begrip der ‘Dimension überhaupt’ en de modale zin-verschuiving in dit pseudo-begrip.
De logicistische zin-verschuivingen zijn in NATORP's poging tot afleiding der zgn. imaginaire getalsfunctie uit de logische grondcorrelatie van afzondering en vereeniging weer met handen te grijpen. Het logicistisch principe van den oorsprong gedoogt niet, dat het theoretisch denken de modale zin-grenzen van getal, ruimte en logische analyse eerbiedigt. De logische denk-continuiteit en denk-richting als ruimte- en bewegingsanalogieën moeten dus dienst doen, om in het getalsbegrip zonder eenig appèl op den originairen modalen zin der ruimte het continuiteits- en dimensiemoment als originair-logische zin-momenten op te nemen.
De multiplicatieve relaties der plus- en minusrichting in de modale tijdsorde van het getal, welke den zin der discrete quantiteit hebben, worden als dimensies geduid en bij aanvaarding van de actueele continuiteit in de grondreeks schijnt dan inderdaad het dimensiebegrip uit den ‘logischen’ zin van het getal afgeleid.
Maar het dimensiebegrip is hier een pseudo-logisch algemeen begrip geworden zonder modale zin-omlijning. Dit blijkt ten overvloede uit NATORP's stelling, dat men, om de dimensies der ruimte te kunnen denken, eerst de ‘Dimensionen überhaupt’ moet kunnen denken.(5)
___________________
(5) a.w. S. 263.
___________________
Het begrip ‘Dimension überhaupt’ is op logistische wijze uit de logische dimensie-analogie gewonnen, waarbij slechts vergeten is, dat deze analogie zonder haar zin-substraat in den originairen modalen ruimte-zin niet kan bestaan.
Intusschen is het voor het inzicht in de complexe structuur der ruimtelijke anticipaties binnen den originairen getalszin zeer leerzaam, dat ook dit logicisme geen kans ziet, het dimensie-moment in het getalsbegrip op te nemen, zonder primair de extensieve continuiteit in de reeks der reeële getalswaarden in te duiden.
Complexe getallenstelsels en groepentheorie. De formalistische opvatting van het symbool i.
In de complexe modale getalsfuncties is inderdaad, gelijk wij reeds vroeger opmerkten, niet een anticipatie op de ruimtelijke dimensies zonder meer, maar veeleer via deze dimensies op de modale bewegingsrichtingen in het spel, en wel onder leiding van de theoretische denk-beweging.
Dit geldt zoowel voor het systeem der zgn. gewone complexe getalsfuncties (A + B i etc.), als voor de systematische uitbreidingen tot de systemen der zgn.
quaternionen, biquaternionen en triquaternionen. De moderne inschakeling van de geheele theorie der complexe getalsfuncties in de zgn. groepentheorie (6), welke de invariante betrekkingen in de transformaties binnen de groep onderzoekt, accentueert slechts dezen stand van zaken, welke de modale structuur-analyse van den getalszin ons onthult.
__________________
(6) Vgl. hierover Dr J. WOLFF, Complexe Getallenstelsels (1917, Groningen), blz. 15 vlg.
__________________
Wanneer inderdaad bij ieder complex getallenstelsel twee verwisselbare groepen van lineaire homogene transformaties behooren en omgekeerd, dan kan het intermodaal zin-verband van de complexe getalsfunctie met de modale structuur der ruimtelijke dimensies en bewegingsrichtingen voor den zin dezer getalsfunctie zelve niet irrelevant zijn. Bij de quaternionen-stelsels, welke uit een reeële en drie imaginaire eenheden (i, j, k) bestaan (7), is reeds het ontbreken van de zgn. commutatieve eigenschap van de vermenigvuldiging, welke ons in de gewone algebra het recht geeft het product ab om te keeren in ba, niet te verstaan zonder verband met de bewegingsrichtingen, welke de quaternion in den zin van het getal anticipeert.
________________
(7) i, j en k worden daarbij geïnterpreteerd als draaiingen van 90° om drie onderling loodrechte assen, zoodat hun kwadraten - 1 zijn.
________________
Het is ook niet zoo, gelijk het formalisme in de wiskunde het voorstelt, dat de imaginaire eenheid i als een zelfgenoegzame abstracte denk-constructie (waarvan men den zin in het midden laat) zich slechts adaequaat zou afbeelden in een zinnelijk ruimtelijk bewegingsbeeld; want wij zullen bij de behandeling der modale subject-object-relatie zien, dat een zinnelijk afbeeldsel een zinnelijk oer-beeld onderstelt en dat een begrip nimmer aanschouwelijk is af te beelden. Het gaat veeleer om een volstrekt a-priorischen inter-modalen zin-samenhang, waarin de complexe getalsfunctie naar haar innerlijke zinstructuur is gevoegd en dien ook het nominalistisch formalisme althans implicile in zijn vermeend willekeurige definities in rekening moet stellen, wil het inderdaad de complexe getalsfuncties in den theoretischen blik fixeeren. (8)
___________________
(8) HANKEL merkt van formalistisch standpunt in zijn Theorie der complexen Zahlensysteme (1867) S. 66 over het symbool i op: ‘es ist weiter nichts als ein Zeichen für ein eingebildetes, mentales Object, welches man die imaginare Einheit nennt, dessen eigentliches Wesen aber in der reinen Theorie ganz unbestimmt bleibt und unbestimmt bleiben musz, da wir uns in dieser nur mit seinen formalen Verknüpfungen zu beschäftigen haben.’ Maar de imaginaire getalsfunctie heeft niet, gelijk H a n k e l meent, een verborgen ‘metaphysisch wezen’. Het gaat om haar functioneele zinstructuur zelve, waarvan haar geheele complex van apriorisch wetmatige betrekkingen afhankelijk is!
___________________
(Herman Dooyeweerd, New Critique of Theoretical Thought, Vol II/ Part I/ Chapt 2/§6 pp 172-174 [De Wijsbegeerte der Wetsidee Deel 2 §5 pp 116-118])